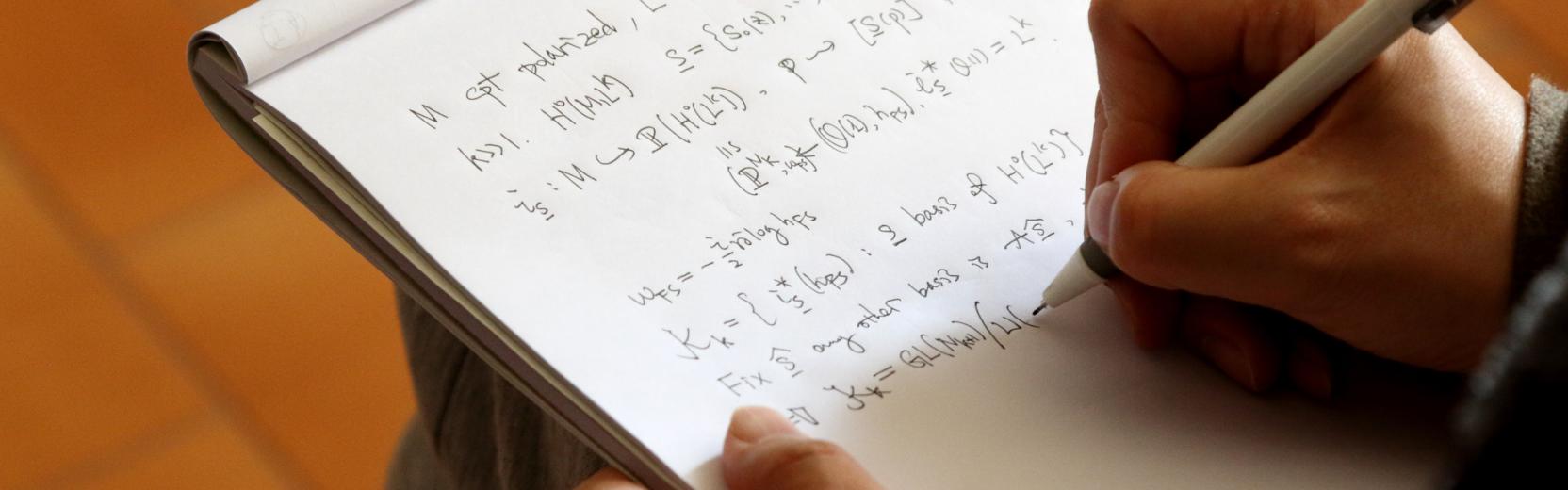Back in the time of Isaac Newton, physicists tried to describe all known phenomena in the Universe through concepts such as force and momentum. Later, with the development of new branches of physics such as thermodynamics, the physical description of nature started to be built around more abstract concepts such as energy and entropy. Nowadays, the frontiers of physics, from quantum computing to cryptography, seem to gravitate around another concept: that of information.
A new paper published in the Journal of Statistical Mechanics explores the connections between the concept of information in thermodynamics and financial markets. 'Information thermodynamics of financial markets: the Glosten–Milgrom model' is the result of a collaboration between ICTP scientist Matteo Marsili of the Centre's Quantitative Life Sciences (QLS) section, Léo Touzo, a master student of École Normale Supérieure, Paris, who joined the QLS Section for his internship, and Don Zagier, holder of the ICTP Ramanujan International Chair and an Emeritus Director at the Max Planck Institute for Mathematics in Bonn, Germany.
"What physicists understand more and more nowadays is that bits of information are a universal currency," says Marsili. "From information thermodynamics, we know that energy can be measured in terms of bits of information, so it's less and less surprising that we can now describe various physical phenomena in terms of information theory. This provides a sort of unified framework to describe many fields of reality."
The starting point of this research is a comparison between a particular kind of financial market and the so-called Szilard engine, that is, the simplest realisation of the Maxwell’s demon idea. This theoretical set-up demonstrates that just the possession of information might in principle have thermodynamic consequences, in a way that one can make a conversion from information to free energy. In a Szilard engine, work can be extracted from a system if a measurement is performed in order to acquire some information from it. The Szilard engine therefore shows that the second law of thermodynamics has to be generalised in cases where some information on the microscopic state of the system is available and can be used to operate on the system.
A principle similar to the second law of thermodynamics applies to financial markets: it is not possible to extract some gain from trading in a financial market, if no side information is available. The Glosten–Milgrom model is a setting that describes a single asset market where a population of traders, both informed and non-informed, interacts with a market maker who sets the prices. This model makes it possible to establish a formal analogy between finance and thermodynamics.
"The main idea is that there might be a broader connection between statistical thermodynamics and finance," says Marsili. "We know from the second law of thermodynamics that you cannot extract free work from a physical system; analogously, in finance we know that there is no free lunch, as you cannot extract money without taking any risk. However, the modified second law of thermodynamics shows that you can extract work from a system if you have some information. Likewise, you can extract money from a financial market if you have some information, and we show in this paper that the maximal gain cannot be larger than the maximal work that one can extract from the analogous thermodynamic system, and we show it in precise mathematical terms."
These precise mathematical terms were implemented and scrupulously tested through the computational skills of Don Zagier, who was involved in the project for his expertise in number theory and his all-around curiosity. "I had never heard of a 'Szilard engine' until I became a co-author of this paper. I had heard of Szilard, of course, and I had heard of engines. And I knew nothing about financial markets, except that there are people who put money in and become very rich or very poor," says Zagier. "We had a very animated email exchange over weeks and months and I was extremely impressed by this idea so I was really excited to contribute to this work."
The idea of mapping one system into another is a traditional idea in physics and mathematics, and it allows to get insights on a certain system from a separate, completely different system. "For example, in our paper we derived the analogue of the thermodynamic temperature for a financial market," says Marsili. "Following the mathematical correspondence between the two systems, we found that it is related to the number of noise traders, which in fact makes a lot of sense. When you only have these noise traders, it means that the market's actors buy and sell at random, so its temperature is infinite."
Even though this work is fundamentally theoretical, there could be situations in which its findings could be applied to real-life practical problems. "The model that we studied could be seen as a game played between one market maker and infinitely many traders," says Marsili. "If we extend our analogy to the case in which the game is played by one market maker and one informed trader, we find that if the trader is infinitely patient, then they can become infinitely rich, though at a rather slow pace. On the other hand, if the trader is impatient, they are going to get a finite amount of money. And this trade-off between how much you gain and how much you wait is a fundamental trade-off in finance. I mean, time is money."
"As I see it, the vision which Matteo had for this paper is much, much broader," says Zagier. "These results are just an example, which happens to be financial markets. But I think the basic point here is that the second law of thermodynamics is not immutable. It's not written in stone. And I felt that the extension of the second law to situations where information is available on the system, which describes a fundamental trade-off between fluctuations and information, is a kind of conceptual idea that may be very broad and may have unexpected applications in domains of science quite different from thermodynamics."
--- Marina Menga